banner



Home  ›  How To Find Acute Angle Of A Right Triangle

How To Find Acute Angle Of A Right Triangle

Written By Monday, May 9, 2022 Add Comment Edit

The Unit Circle: Sine and Cosine Functions

Right Triangle Trigonometry

Learning Objectives

In this department you will:

Mt. Everest, which straddles the edge between Red china and Nepal, is the tallest mountain in the world. Measuring its peak is no piece of cake task and, in fact, the actual measurement has been a source of controversy for hundreds of years. The measurement procedure involves the use of triangles and a branch of mathematics known as trigonometry. In this section, we will ascertain a new group of functions known as trigonometric functions, and observe out how they tin can be used to measure heights, such as those of the tallest mountains.

Using Right Triangles to Evaluate Trigonometric Functions

(Figure) shows a right triangle with a vertical side of length\,y\,and a horizontal side has length\,x.\,Notice that the triangle is inscribed in a circle of radius ane. Such a circle, with a center at the origin and a radius of 1, is known equally a unit circumvolve.

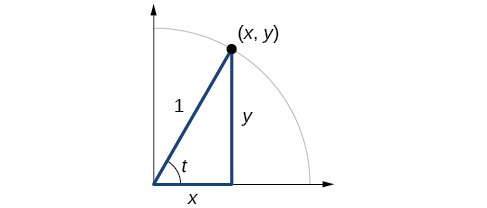
Graph of quarter circle with radius of 1. Inscribed triangle with an angle of t. Point of (x,y) is at intersection of terminal side of angle and edge of circle.
Figure 1.

We can ascertain the trigonometric functions in terms an angle t and the lengths of the sides of the triangle. The adjacent side is the side closest to the angle, 10. (Adjacent means "adjacent to.") The reverse side is the side across from the bending, y. The hypotenuse is the side of the triangle opposite the right angle, 1. These sides are labeled in (Figure).

Given a right triangle with an acute angle of\,t,the first iii trigonometric functions are listed.

\begin{array}{ccc}\phantom{\rule{2.5em}{0ex}}\text{Sine}\hfill & \phantom{\rule{1em}{0ex}}\text{sin }t\hfill & =\frac{\text{opposite}}{\text{hypotenuse}}\hfill \end{array}

\begin{array}{ccc}\phantom{\rule{1.5em}{0ex}}\text{Cosine}\hfill & \phantom{\rule{1em}{0ex}}\text{cos }t\hfill & =\frac{\text{adjacent}}{\text{hypotenuse}}\hfill \end{array}

\begin{array}{ccc}\hfill \text{Tangent}& \phantom{\rule{1em}{0ex}}\text{tan }t\hfill & =\frac{\text{opposite}}{\text{adjacent}}\hfill \end{array}

A common mnemonic for remembering these relationships is SohCahToa, formed from the first letters of " South ine is o pposite over h ypotenuse, C osine is a djacent over h ypotenuse, T angent is o pposite over a djacent."

For the triangle shown in (Figure), nosotros have the following.

\begin{array}{ccc}\hfill \text{sin }t& =& \frac{y}{1}\hfill \\ \hfill \text{cos }t& =& \frac{x}{1}\hfill \\ \hfill \text{tan }t& =& \frac{y}{x}\hfill \end{array}

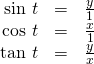
How To

Given the side lengths of a correct triangle and i of the acute angles, discover the sine, cosine, and tangent of that angle.

  1. Find the sine as the ratio of the opposite side to the hypotenuse.
  2. Discover the cosine every bit the ratio of the adjacent side to the hypotenuse.
  3. Detect the tangent every bit the ratio of the opposite side to the adjacent side.

Evaluating a Trigonometric Office of a Correct Triangle

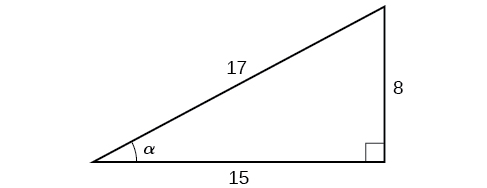
Given the triangle shown in (Effigy), find the value of\,\mathrm{cos}\,\alpha .

A right triangle with side lengths of 8, 15, and 17. Angle alpha also labeled which is opposite to the side labeled 8.
Effigy 3.

[reveal-answer q="fs-id2159428″]Show Solution[/reveal-respond]
[hidden-answer a="fs-id2159428″]

The side adjacent to the angle is 15, and the hypotenuse of the triangle is 17.

\begin{array}{ccc}\hfill \mathrm{cos}\left(\alpha \right)& =& \frac{\text{adjacent}}{\text{hypotenuse}}\hfill \\ & =& \frac{15}{17}\hfill \end{array}[/hidden-reply]

Try Information technology

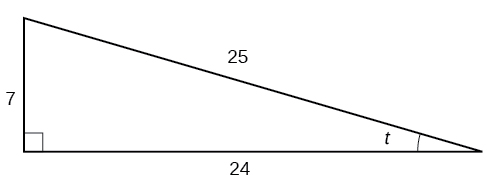
Given the triangle shown in (Effigy), detect the value of\,\text{sin}\,t.

A right triangle with sides of 7, 24, and 25. Also labeled is angle t which is opposite the side labeled 7.
Figure iv.

[reveal-answer q="1590112″]Show Solution[/reveal-reply][hidden-answer a="1590112″]
\frac{7}{25}[/hidden-answer]

Reciprocal Functions

In addition to sine, cosine, and tangent, there are three more functions. These too are divers in terms of the sides of the triangle.

\begin{array}{ccc}\phantom{\rule{2em}{0ex}}\text{Secant}\hfill & \phantom{\rule{1em}{0ex}}\text{sec }t\hfill & =\frac{\text{hypotenuse}}{\text{adjacent}}\hfill \end{array}

\begin{array}{ccc}\hfill \phantom{\rule{1.3em}{0ex}}\text{Cosecant}& \hfill \phantom{\rule{1em}{0ex}}\text{csc }t& =\frac{\text{hypotenuse}}{\text{opposite}}\hfill \end{array}

\begin{array}{ccc}\hfill \text{Cotangent}& \phantom{\rule{1em}{0ex}}\text{cot }t& =\frac{\text{adjacent}}{\text{opposite}}\hfill \end{array}

Take another expect at these definitions. These functions are the reciprocals of the first three functions.

\begin{array}{cccccc}\hfill \text{sin }t& =& \frac{1}{\text{csc }t}\hfill & \hfill \phantom{\rule{2em}{0ex}}\text{csc }t& =& \frac{1}{\text{sin }t}\hfill \\ \hfill \text{cos }t& =& \frac{1}{\text{sec }t}\hfill & \hfill \phantom{\rule{2em}{0ex}}\text{sec }t& =& \frac{1}{\text{cos }t}\hfill \\ \hfill \text{tan }t& =& \frac{1}{\text{cot }t}\hfill & \hfill \phantom{\rule{2em}{0ex}}\text{cot }t& =& \frac{1}{\text{tan }t}\hfill \end{array}

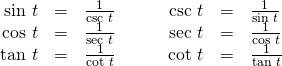
When working with right triangles, continue in heed that the same rules apply regardless of the orientation of the triangle. In fact, we can evaluate the six trigonometric functions of either of the two acute angles in the triangle in (Figure). The side opposite one acute bending is the side adjacent to the other acute angle, and vice versa.

Right triangle with angles alpha and beta. Sides are labeled hypotenuse, adjacent to alpha/opposite to beta, and adjacent to beta/opposite alpha.
Figure 5. The side next to 1 angle is opposite the other angle.

Many problems inquire for all half dozen trigonometric functions for a given angle in a triangle. A possible strategy to utilise is to observe the sine, cosine, and tangent of the angles first. And so, find the other trigonometric functions easily using the reciprocals.

How To

Given the side lengths of a right triangle, evaluate the six trigonometric functions of i of the astute angles.

  1. If needed, draw the right triangle and label the angle provided.
  2. Place the angle, the adjacent side, the side contrary the angle, and the hypotenuse of the right triangle.
  3. Find the required function:
    • sine as the ratio of the opposite side to the hypotenuse
    • cosine as the ratio of the adjacent side to the hypotenuse
    • tangent every bit the ratio of the opposite side to the adjacent side
    • secant as the ratio of the hypotenuse to the adjacent side
    • cosecant as the ratio of the hypotenuse to the opposite side
    • cotangent as the ratio of the adjacent side to the contrary side

Evaluating Trigonometric Functions of Angles Not in Standard Position

Using the triangle shown in (Figure), evaluate\,\mathrm{sin}\,\alpha ,\mathrm{cos}\,\alpha ,\mathrm{tan}\,\alpha ,\mathrm{sec}\,\alpha ,\mathrm{csc}\,\alpha ,\text{and}\,\mathrm{cot}\,\alpha .

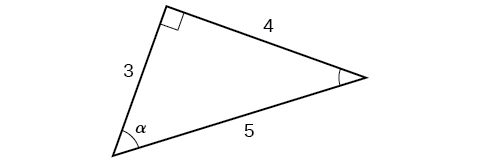
Right triangle with sides of 3, 4, and 5. Angle alpha is also labeled which is opposite the side labeled 4.
Effigy 6.

[reveal-answer q="2211290″]Show Solution[/reveal-answer][hidden-answer a="2211290″]

\begin{array}{ccc}\hfill \text{sin }\alpha & =\frac{\text{opposite }\alpha }{\text{hypotenuse}}\hfill & =\frac{4}{5}\hfill \\ \hfill \text{cos }\alpha & =\frac{\text{adjacent to }\alpha }{\text{hypotenuse}}\hfill & =\frac{3}{5}\hfill \\ \hfill \text{tan }\alpha & =\frac{\text{opposite }\alpha }{\text{adjacent to }\alpha }\hfill & =\frac{4}{3}\hfill \\ \hfill \text{sec }\alpha & =\frac{\text{hypotenuse}}{\text{adjacent to }\alpha }\hfill & =\frac{5}{3}\hfill \\ \hfill \text{csc }\alpha & =\frac{\text{hypotenuse}}{\text{opposite }\alpha }\hfill & =\frac{5}{4}\hfill \\ \hfill \text{cot }\alpha & =\frac{\text{adjacent to }\alpha }{\text{opposite }\alpha }\hfill & =\frac{3}{4}\hfill \end{array}[/subconscious-answer]

Analysis

Another approach would have been to observe sine, cosine, and tangent showtime. Then notice their reciprocals to determine the other functions.

\text{sec }\alpha =\frac{1}{\text{cos }\alpha }=\frac{1}{\frac{3}{5}}=\frac{5}{3}

\text{csc }\alpha =\frac{1}{\text{csc }\alpha }=\frac{1}{\frac{4}{5}}=\frac{5}{4}

\text{cot }\alpha =\frac{1}{\text{tan }\alpha }=\frac{1}{\frac{4}{3}}=\frac{3}{4}

Try It

Using the triangle shown in (Effigy),evaluate\,\text{sin}\,t,\text{cos}\,t,\text{tan}\,t,\text{sec}\,t,\text{csc}\,t,\text{and}\,\text{cot}\,t.

Right triangle with sides 33, 56, and 65. Angle t is also labeled which is opposite to the side labeled 33.
Figure 7.

[reveal-answer q="fs-id1482962″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1482962″]

\begin{array}{ccccccccc}\hfill \text{sin }t& =& \frac{33}{65},\hfill & \hfill \text{cos }t& =& \frac{56}{65},\hfill & \hfill \text{tan }t& =& \frac{33}{56},\hfill \\ \hfill \text{sec }t& =& \frac{65}{56},\hfill & \hfill \text{csc }t& =& \frac{65}{33},\hfill & \hfill \text{cot }t& =& \frac{56}{33}\hfill \end{array}

[/hidden-answer]

Finding Trigonometric Functions of Special Angles Using Side Lengths

It is helpful to evaluate the trigonometric functions every bit they relate to the special angles—multiples of\,30°,60°,and\,45°.\,Recall, notwithstanding, that when dealing with right triangles, we are limited to angles betwixt\,0°\text{ and 90°}\text{.}

Suppose nosotros have a\,30°,60°,90°\,triangle, which can also be described every bit a\,\frac{\pi }{6},\frac{\pi }{3},\frac{\pi }{2}\,triangle. The sides take lengths in the relation\,s,\sqrt{3}s,2s.\,The sides of a\,45°,45°,90°\,triangle, which can also exist described every bit a\,\frac{\pi }{4},\frac{\pi }{4},\frac{\pi }{2}\,triangle, have lengths in the relation\,s,s,\sqrt{2}s.\,These relations are shown in (Effigy).

Two side-by-side graphs of circles with inscribed angles. First circle has angle of pi/3 inscribed, radius of 2s, base of length s and height of length . Second circle has angle of pi/4 inscribed with radius , base of length s and height of length s.
Figure viii. Side lengths of special triangles

Nosotros tin then use the ratios of the side lengths to evaluate trigonometric functions of special angles.

How To

Given trigonometric functions of a special angle, evaluate using side lengths.

  1. Utilise the side lengths shown in (Figure) for the special angle you wish to evaluate.
  2. Use the ratio of side lengths appropriate to the function you lot wish to evaluate.

Evaluating Trigonometric Functions of Special Angles Using Side Lengths

Detect the exact value of the trigonometric functions of\,\frac{\pi }{3},using side lengths.

[reveal-reply q="1736320″]Show Solution[/reveal-answer][hidden-answer a="1736320″]

\begin{array}{ccccc}\hfill \mathrm{sin}\left(\frac{\pi }{3}\right)& =\frac{\text{opp}}{\text{hyp}}\hfill & =\frac{\sqrt{3s}}{2s}\hfill & =\frac{\sqrt{3}}{2}\hfill & \\ \hfill \mathrm{cos}\left(\frac{\pi }{3}\right)& =\frac{\text{adj}}{\text{hyp}}\hfill & =\frac{s}{2s}\hfill & =\frac{1}{2}\hfill & \\ \hfill \mathrm{tan}\left(\frac{\pi }{3}\right)& =\frac{\text{opp}}{\text{adj}}\hfill & =\frac{\sqrt{3}s}{s}\hfill & =\sqrt{3}\hfill & \\ \hfill \mathrm{sec}\left(\frac{\pi }{3}\right)& =\frac{\text{hyp}}{\text{adj}}\hfill & =\frac{2s}{s}\hfill & =2\hfill & \\ \hfill \mathrm{csc}\left(\frac{\pi }{3}\right)& =\frac{\text{hyp}}{\text{opp}}\hfill & =\frac{2s}{\sqrt{3}s}\hfill & =\frac{2}{\sqrt{3}}\hfill & =\frac{2\sqrt{3}}{3}\hfill \\ \hfill \mathrm{cot}\left(\frac{\pi }{3}\right)& =\frac{\text{adj}}{\text{opp}}\hfill & =\frac{s}{\sqrt{3}s}\hfill & =\frac{1}{\sqrt{3}}\hfill & =\frac{\sqrt{3}}{3}\hfill \end{array}[/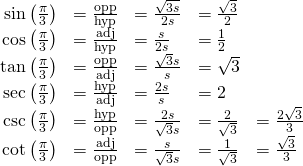subconscious-answer]

Endeavour It

Observe the exact value of the trigonometric functions of\,\frac{\pi }{4},using side lengths.

[reveal-answer q="fs-id1590664″]Show Solution[/reveal-reply]
[hidden-answer a="fs-id1590664″]

\begin{array}{ccc}\hfill \text{sin}\left(\frac{\pi }{4}\right)& =& \frac{\sqrt{2}}{2},\text{cos}\left(\frac{\pi }{4}\right)=\frac{\sqrt{2}}{2},\mathrm{tan}\left(\frac{\pi }{4}\right)=1,\hfill \\ \hfill \text{sec}\left(\frac{\pi }{4}\right)& =& \sqrt{2},\text{csc}\left(\frac{\pi }{4}\right)=\sqrt{2},\text{cot}\left(\frac{\pi }{4}\right)=1\hfill \end{array}

[/subconscious-answer]

Using Equal Cofunction of Complements

If we await more closely at the relationship between the sine and cosine of the special angles, nosotros observe a blueprint. In a right triangle with angles of\,\frac{\pi }{6}\,and\,\frac{\pi }{3},we see that the sine of\,\frac{\pi }{3},namely\,\frac{\sqrt{3}}{2},is also the cosine of\,\frac{\pi }{6},while the sine of\,\frac{\pi }{6},namely\,\frac{1}{2},is also the cosine of\,\frac{\pi }{3}.

\begin{array}{cccc}\hfill \mathrm{sin}\frac{\pi }{3}& =\mathrm{cos}\frac{\pi }{6}\hfill & =\frac{\sqrt{3}s}{2s}\hfill & =\frac{\sqrt{3}}{2}\hfill \\ \hfill \mathrm{sin}\frac{\pi }{6}& =\mathrm{cos}\frac{\pi }{3}\hfill & =\frac{s}{2s}\hfill & =\frac{1}{2}\hfill \end{array}

See (Effigy).

This result should not be surprising considering, equally we see from (Effigy), the side opposite the bending of\,\frac{\pi }{3}\,is as well the side adjacent to\,\frac{\pi }{6},so\,\mathrm{sin}\left(\frac{\pi }{3}\right)\,and\,\mathrm{cos}\left(\frac{\pi }{6}\right)\,are exactly the same ratio of the same two sides,\,\sqrt{3}s\,and\,2s.\,Similarly,\,\mathrm{cos}\left(\frac{\pi }{3}\right)\,and\,\mathrm{sin}\left(\frac{\pi }{6}\right)\,are as well the aforementioned ratio using the aforementioned two sides,\,s\,and\,2s.

The interrelationship between the sines and cosines of\,\frac{\pi }{6}\,and\,\frac{\pi }{3}\,also holds for the two acute angles in any right triangle, since in every case, the ratio of the same two sides would constitute the sine of one angle and the cosine of the other. Since the iii angles of a triangle add to\,\pi ,and the right bending is\,\frac{\pi }{2},the remaining 2 angles must as well add upward to\,\frac{\pi }{2}.\,That means that a right triangle can exist formed with any two angles that add to\,\frac{\pi }{2}\,—in other words, any 2 complementary angles. So we may state a cofunction identity: If any two angles are complementary, the sine of one is the cosine of the other, and vice versa. This identity is illustrated in (Figure).

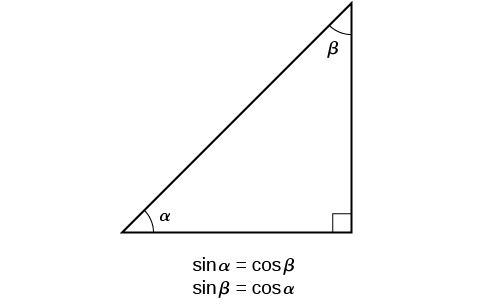
Right triangle with angles alpha and beta. Equivalence between sin alpha and cos beta. Equivalence between sin beta and cos alpha.
Figure x. Cofunction identity of sine and cosine of complementary angles

Using this identity, nosotros tin can state without calculating, for instance, that the sine of\,\frac{\pi }{12}\,equals the cosine of\,\frac{5\pi }{12},and that the sine of\,\frac{5\pi }{12}\,equals the cosine of\,\frac{\pi }{12}.\,Nosotros tin also state that if, for a given angle\,t,\mathrm{cos}\text{ }t=\frac{5}{13},then\,\mathrm{sin}\left(\frac{\pi }{2}-t\right)=\frac{5}{13}\,as well.

How To

Given the sine and cosine of an bending, find the sine or cosine of its complement.

  1. To detect the sine of the complementary angle, find the cosine of the original angle.
  2. To find the cosine of the complementary bending, discover the sine of the original angle.

Using Cofunction Identities

If\,\mathrm{sin}\,t=\frac{5}{12},discover\,\mathrm{cos}\left(\frac{\pi }{2}-t\right).

[reveal-answer q="2026175″]Show Solution[/reveal-answer][hidden-answer a="2026175″]

Co-ordinate to the cofunction identities for sine and cosine, we have the following.

\mathrm{sin}\,t=\mathrm{cos}\,\left(\frac{\pi }{2}-t\right)

So

\mathrm{cos}\,\left(\frac{\pi }{2}-t\right)=\frac{5}{12}[/hidden-answer]

Try It

If\,\mathrm{csc}\,\left(\frac{\pi }{6}\right)=2,detect\,\mathrm{sec}\,\left(\frac{\pi }{3}\right).

[reveal-answer q="fs-id1551183″]Show Solution[/reveal-answer]
[hidden-respond a="fs-id1551183″]

2

[/hidden-answer]

Using Trigonometric Functions

In previous examples, nosotros evaluated the sine and cosine in triangles where we knew all 3 sides. But the existent power of right-triangle trigonometry emerges when we wait at triangles in which nosotros know an angle but do not know all the sides.

How To

Given a correct triangle, the length of one side, and the measure out of one acute angle, find the remaining sides.

  1. For each side, select the trigonometric function that has the unknown side as either the numerator or the denominator. The known side will in turn be the denominator or the numerator.
  2. Write an equation setting the function value of the known angle equal to the ratio of the corresponding sides.
  3. Using the value of the trigonometric role and the known side length, solve for the missing side length.

Finding Missing Side Lengths Using Trigonometric Ratios

Find the unknown sides of the triangle in (Figure).

A right triangle with sides a, c, and 7. Angle of 30 degrees is also labeled which is opposite the side labeled 7.
Figure xi.

[reveal-reply q="fs-id1498470″]Bear witness Solution[/reveal-answer]
[hidden-respond a="fs-id1498470″]

We know the angle and the reverse side, and so nosotros tin employ the tangent to find the adjacent side.

\mathrm{tan}\left(30°\right)=\frac{7}{a}

We rearrange to solve for\,a.

\begin{array}{ccc}\hfill a& =& \frac{7}{\mathrm{tan}\left(30°\right)}\hfill \\ & \approx & 12.1\hfill \end{array}

Nosotros can utilize the sine to observe the hypotenuse.

\mathrm{sin}\left(30°\right)=\frac{7}{c}

Again, we rearrange to solve for\,c.

\begin{array}{ccc}\hfill c& =& \frac{7}{\mathrm{sin}\left(30°\right)}\hfill \\ & =& 14\hfill \end{array}[/hidden-reply]

Attempt It

A right triangle has 1 bending of\,\frac{\pi }{3}\,and a hypotenuse of 20. Detect the unknown sides and angle of the triangle.

[reveal-answer q="fs-id2353470″]Show Solution[/reveal-answer]
[hidden-respond a="fs-id2353470″]

\text{adjacent}=10;\text{opposite}=10\sqrt{3};missing angle is\,\frac{\pi }{6}

[/hidden-answer]

Using Correct Triangle Trigonometry to Solve Applied Problems

Correct-triangle trigonometry has many practical applications. For example, the power to compute the lengths of sides of a triangle makes it possible to find the height of a tall object without climbing to the top or having to extend a tape mensurate along its pinnacle. Nosotros do so by measuring a distance from the base of the object to a point on the ground some distance abroad, where we can look upward to the acme of the tall object at an bending. The bending of elevation of an object above an observer relative to the observer is the angle between the horizontal and the line from the object to the observer'due south eye. The right triangle this position creates has sides that represent the unknown height, the measured distance from the base, and the angled line of sight from the ground to the top of the object. Knowing the measured distance to the base of the object and the angle of the line of sight, we can utilize trigonometric functions to calculate the unknown height.

Similarly, we tin can class a triangle from the top of a alpine object by looking downward. The bending of low of an object below an observer relative to the observer is the angle betwixt the horizontal and the line from the object to the observer'south eye. See (Figure).

Diagram of a radio tower with line segments extending from the top and base of the tower to a point on the ground some distance away. The two lines and the tower form a right triangle. The angle near the top of the tower is the angle of depression. The angle on the ground at a distance from the tower is the angle of elevation.
Figure 12.

How To

Given a tall object, measure its height indirectly.

  1. Make a sketch of the trouble situation to keep track of known and unknown information.
  2. Lay out a measured altitude from the base of the object to a betoken where the elevation of the object is clearly visible.
  3. At the other terminate of the measured distance, expect up to the top of the object. Measure the bending the line of sight makes with the horizontal.
  4. Write an equation relating the unknown summit, the measured altitude, and the tangent of the bending of the line of sight.
  5. Solve the equation for the unknown height.

Measuring a Distance Indirectly

To discover the acme of a tree, a person walks to a signal 30 anxiety from the base of the tree. She measures an bending of\,57°\,between a line of sight to the top of the tree and the ground, as shown in (Figure). Find the height of the tree.

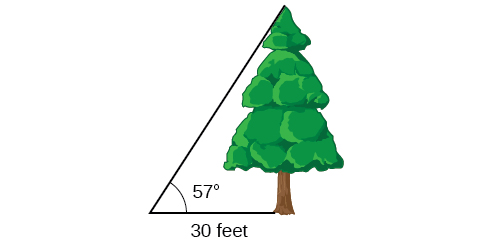
A tree with angle of 57 degrees from vantage point. Vantage point is 30 feet from tree.
Effigy thirteen.

[reveal-respond q="fs-id2189191″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id2189191″]

Nosotros know that the angle of superlative is\,57°\,and the adjacent side is 30 ft long. The opposite side is the unknown elevation.

The trigonometric function relating the side contrary to an angle and the side adjacent to the angle is the tangent. So we will country our information in terms of the tangent of\,57°,letting\,h\,exist the unknown height.

\begin{array}{cccc}\hfill \text{tan }\theta & =& \frac{\text{opposite}}{\text{adjacent}}\hfill & \\ \hfill \text{tan}\left(57°\right)& =& \frac{h}{30}\hfill & \phantom{\rule{1em}{0ex}}\text{Solve for }h.\hfill \\ \hfill h& =& \text{30tan}\left(\text{57°}\right)\hfill & \phantom{\rule{1em}{0ex}}\text{Multiply}\text{.}\hfill \\ \hfill h& \approx & 46.2\hfill & \phantom{\rule{1em}{0ex}}\text{Use a calculator}\text{.}\hfill \end{array}

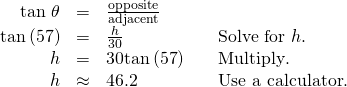
The tree is approximately 46 feet alpine.[/hidden-answer]

Try It

How long a ladder is needed to accomplish a windowsill 50 feet above the ground if the ladder rests against the building making an angle of\,\frac{5\pi }{12}\,with the ground? Round to the nearest foot.

[reveal-answer q="fs-id2128793″]Evidence Solution[/reveal-answer]
[hidden-answer a="fs-id2128793″]

Almost 52 ft

[/subconscious-reply]

Fundamental Concepts

  • Nosotros tin define trigonometric functions as ratios of the side lengths of a correct triangle. See (Figure).
  • The same side lengths can be used to evaluate the trigonometric functions of either astute bending in a right triangle. Run across (Figure).
  • We can evaluate the trigonometric functions of special angles, knowing the side lengths of the triangles in which they occur. Meet (Figure).
  • Any two complementary angles could be the 2 acute angles of a right triangle.
  • If ii angles are complementary, the cofunction identities state that the sine of i equals the cosine of the other and vice versa. Run into (Effigy).
  • We can use trigonometric functions of an angle to find unknown side lengths.
  • Select the trigonometric role representing the ratio of the unknown side to the known side. Come across (Figure).
  • Right-triangle trigonometry facilitates the measurement of inaccessible heights and distances.
  • The unknown height or distance can be found by creating a right triangle in which the unknown pinnacle or distance is ane of the sides, and another side and angle are known. Meet (Effigy).

Section Exercises

Verbal

For the given right triangle, characterization the adjacent side, opposite side, and hypotenuse for the indicated angle.

A right triangle.

[reveal-respond q="fs-id2133705″]Prove Solution[/reveal-reply]
[hidden-reply a="fs-id2133705″]A right triangle with side opposite, adjacent, and hypotenuse labeled.[/hidden-reply]

When a right triangle with a hypotenuse of 1 is placed in a circumvolve of radius 1, which sides of the triangle correspond to the x– and y-coordinates?

The tangent of an bending compares which sides of the right triangle?

[reveal-answer q="fs-id2546065″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id2546065″]

The tangent of an angle is the ratio of the opposite side to the adjacent side.

[/hidden-answer]

What is the relationship between the two acute angles in a correct triangle?

Explain the cofunction identity.

[reveal-reply q="fs-id1367073″]Bear witness Solution[/reveal-reply]
[subconscious-answer a="fs-id1367073″]

For case, the sine of an angle is equal to the cosine of its complement; the cosine of an angle is equal to the sine of its complement.

[/hidden-answer]

Algebraic

For the following exercises, apply cofunctions of complementary angles.

\mathrm{cos}\left(34°\right)=\mathrm{sin}\left(\_\_\_°\right)

\mathrm{cos}\left(\frac{\pi }{3}\right)=\mathrm{sin}\left(\_\_\_\right)

[reveal-reply q="fs-id2674305″]Bear witness Solution[/reveal-answer]
[hidden-answer a="fs-id2674305″]

\frac{\pi }{6}

[/hidden-answer]

\mathrm{csc}\left(21°\right)=\mathrm{sec}\left(\_\_\_°\right)

\mathrm{tan}\left(\frac{\pi }{4}\right)=\mathrm{cot}\left(\_\_\_\right)

[reveal-answer q="fs-id932114″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id932114″]

\frac{\pi }{4}

[/hidden-answer]

For the following exercises, detect the lengths of the missing sides if side\,a\,is contrary bending\,A,side\,b\,is opposite bending\,B,and side\,c\,is the hypotenuse.

\mathrm{cos}\,B=\frac{4}{5},a=10

\mathrm{sin}\,B=\frac{1}{2},a=20

[reveal-respond q="fs-id2196577″]Prove Solution[/reveal-reply]
[hidden-answer a="fs-id2196577″]

b=\frac{20\sqrt{3}}{3},c=\frac{40\sqrt{3}}{3}

[/hidden-respond]

\mathrm{tan}\,A=\frac{5}{12},b=6

\mathrm{tan}\,A=100,b=100

[reveal-respond q="fs-id1714556″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1714556″]

a=10,000,c=10,00.5

[/hidden-reply]

\mathrm{sin}\,B=\frac{1}{\sqrt{3}},a=2

a=5,\measuredangle \,A=60°

[reveal-answer q="fs-id1596950″]Bear witness Solution[/reveal-reply]
[hidden-answer a="fs-id1596950″]

b=\frac{5\sqrt{3}}{3},c=\frac{10\sqrt{3}}{3}

[/hidden-answer]

c=12,\measuredangle \,A=45°

Graphical

For the following exercises, employ (Effigy) to evaluate each trigonometric function of angle\,A.

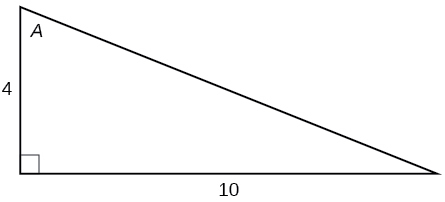
A right triangle with sides 4 and 10 and angle of A labeled which is opposite the side labeled 10.
Figure fourteen.

\mathrm{sin}\,A

[reveal-answer q="fs-id1450359″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id1450359″]

\frac{5\sqrt{29}}{29}

[/subconscious-answer]

\mathrm{cos}\,A

\mathrm{tan}\,A

[reveal-answer q="2673682″]Show Solution[/reveal-respond][subconscious-answer a="2673682″]
\frac{5}{2}[/hidden-respond]

\mathrm{csc}\,A

\mathrm{sec}\,A

[reveal-answer q="fs-id2674056″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id2674056″]

\frac{\sqrt{29}}{2}

[/subconscious-answer]

\mathrm{cot}\,A

For the following exercises, utilise (Effigy) to evaluate each trigonometric office of angle\,A.

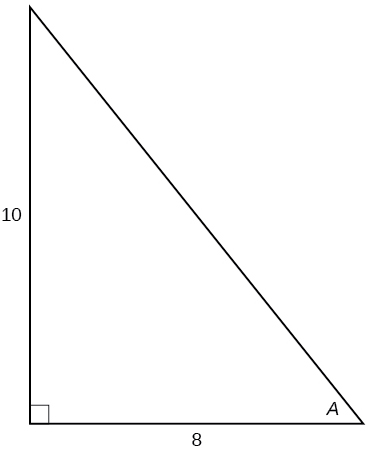
A right triangle with sides of 10 and 8 and angle of A labeled which is opposite the side labeled 10.
Figure 15.

\mathrm{sin}\,A

[reveal-respond q="fs-id2363602″]Testify Solution[/reveal-answer]
[hidden-answer a="fs-id2363602″]

\frac{5\sqrt{41}}{41}

[/hidden-reply]

\mathrm{cos}\,A

\mathrm{tan}\,A

[reveal-reply q="fs-id2256725″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id2256725″]

\frac{5}{4}

[/hidden-respond]

\mathrm{csc}\,A

\mathrm{sec}\,A

[reveal-answer q="fs-id2027628″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id2027628″]

\frac{\sqrt{41}}{4}

[/subconscious-respond]

\mathrm{cot}\,A

For the following exercises, solve for the unknown sides of the given triangle.

A right triangle with sides of 7, b, and c labeled. Angles of B and 30 degrees also labeled. The 30 degree angle is opposite the side labeled 7.

[reveal-respond q="fs-id1504623″]Evidence Solution[/reveal-answer]
[subconscious-answer a="fs-id1504623″]

c=14,b=7\sqrt{3}

[/hidden-answer]

A right triangle with sides of 10, a, and c. Angles of 60 degrees and A also labeled. The 60 degree angle is opposite the side labeled 10.

A right triangle with corners labeled A, B, and C. Hypotenuse has length of 15 times square root of 2. Angle B is 45 degrees.

[reveal-answer q="fs-id2240073″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id2240073″]

a=15,b=15

[/hidden-answer]

Engineering science

For the following exercises, use a reckoner to detect the length of each side to four decimal places.

A right triangle with sides of 10, a, and c. Angles of A and 62 degrees are also labeled. The 62 degree angle is opposite the side labeled 10.

A right triangle with sides of 7, b, and c. Angles of 35 degrees and B are also labeled.

[reveal-respond q="fs-id2566188″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id2566188″]

b=9.9970,c=12.2041

[/hidden-answer]

A right triangle with sides of a, b, and 10 labeled. Angles of 65 degrees and B are also labeled.

A right triangle with sides a, b, and 12. Angles of 10 degrees and B are also labeled.

[reveal-respond q="fs-id2513969″]Show Solution[/reveal-answer]
[subconscious-answer a="fs-id2513969″]

a=2.0838,b=11.8177

[/hidden-answer]

A right triangle with corners labeled A, B, and C. Sides labeled b, c, and 16.5. Angle of 81 degrees also labeled.

b=15,\measuredangle \,B=15°

[reveal-respond q="fs-id2571997″]Prove Solution[/reveal-respond]
[hidden-answer a="fs-id2571997″]

a=55.9808,c=57.9555

[/hidden-answer]

c=200,\measuredangle \,B=5°

c=50,\measuredangle \,B=21°

[reveal-answer q="fs-id2251440″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id2251440″]

a=46.6790,b=17.9184

[/hidden-reply]

a=30,\measuredangle \,A=27°

b=3.5,\measuredangle \,A=78°

[reveal-reply q="fs-id2248439″]Show Solution[/reveal-respond]
[hidden-respond a="fs-id2248439″]

a=16.4662,c=16.8341

[/hidden-answer]

Extensions

Observe\,x.

A triangle with angles of 63 degrees and 39 degrees and side x. Bisector in triangle with length of 82.

Observe\,x.

A triangle with angles of 36 degrees and 50 degrees and side x. Bisector in triangle with length of 85.

[reveal-reply q="fs-id2084785″]Show Solution[/reveal-answer]
[hidden-respond a="fs-id2084785″]

188.3159

[/hidden-answer]

Detect\,x.

A right triangle with side of 115 and angle of 35 degrees. Within right triangle there is another right triangle with angle of 56 degrees. Side length difference between two triangles is x.

Find\,x.

A right triangle with side of 119 and angle of 26 degrees. Within right triangle there is another right triangle with angle of 70 degrees instead of 26 degrees. Difference in side length between two triangles is x.

[reveal-answer q="fs-id1307785″]Bear witness Solution[/reveal-reply]
[hidden-answer a="fs-id1307785″]

200.6737

[/subconscious-answer]

A radio tower is located 400 feet from a building. From a window in the building, a person determines that the bending of top to the top of the tower is\,36°,and that the angle of depression to the bottom of the belfry is\,23°.\,How tall is the tower?

A radio tower is located 325 feet from a building. From a window in the edifice, a person determines that the bending of elevation to the meridian of the tower is\,43°,and that the angle of low to the lesser of the tower is\,31°.\,How tall is the tower?

[reveal-answer q="fs-id2160790″]Show Solution[/reveal-reply]
[hidden-answer a="fs-id2160790″]

498.3471 ft

[/subconscious-answer]

A 200-foot tall monument is located in the distance. From a window in a building, a person determines that the angle of height to the height of the monument is\,15°,and that the angle of low to the bottom of the monument is\,2°.\,How far is the person from the monument?

A 400-foot alpine monument is located in the distance. From a window in a building, a person determines that the angle of elevation to the top of the monument is\,18°,and that the angle of depression to the bottom of the monument is\,3°.\,How far is the person from the monument?

[reveal-answer q="fs-id2189404″]Show Solution[/reveal-respond]
[hidden-answer a="fs-id2189404″]

1060.09 ft

[/hidden-respond]

There is an antenna on the superlative of a building. From a location 300 anxiety from the base of the building, the bending of meridian to the top of the edifice is measured to be\,40°.\,From the same location, the bending of meridian to the summit of the antenna is measured to be\,43°.\,Observe the height of the antenna.

In that location is lightning rod on the top of a building. From a location 500 feet from the base of the building, the bending of pinnacle to the meridian of the building is measured to be\,36°.\,From the same location, the angle of peak to the meridian of the lightning rod is measured to be\,38°.\,Find the height of the lightning rod.

[reveal-answer q="fs-id2165385″]Evidence Solution[/reveal-reply]
[hidden-answer a="fs-id2165385″]

27.372 ft

[/subconscious-answer]

Existent-World Applications

A 33-ft ladder leans confronting a building so that the bending betwixt the footing and the ladder is\,80°.\,How high does the ladder reach up the side of the edifice?

A 23-ft ladder leans confronting a building and so that the angle between the ground and the ladder is\,80°.\,How loftier does the ladder reach upward the side of the building?

[reveal-answer q="fs-id2204154″]Testify Solution[/reveal-respond]
[subconscious-answer a="fs-id2204154″]

22.6506 ft

[/hidden-answer]

The angle of elevation to the top of a edifice in New York is found to be 9 degrees from the ground at a distance of 1 mile from the base of operations of the edifice. Using this information, discover the top of the building.

The bending of height to the top of a building in Seattle is constitute to be 2 degrees from the ground at a altitude of 2 miles from the base of operations of the building. Using this data, discover the top of the building.

[reveal-reply q="fs-id2528007″]Show Solution[/reveal-answer]
[hidden-answer a="fs-id2528007″]

368.7633 ft

[/subconscious-answer]

Bold that a 370-foot tall giant redwood grows vertically, if I walk a sure altitude from the tree and mensurate the angle of elevation to the pinnacle of the tree to be\,60°,how far from the base of the tree am I?

Glossary

adjacent side
in a right triangle, the side between a given angle and the right bending
angle of depression
the angle between the horizontal and the line from the object to the observer's middle, assuming the object is positioned lower than the observer
angle of elevation
the bending between the horizontal and the line from the object to the observer's center, assuming the object is positioned higher than the observer
opposite side
in a right triangle, the side most distant from a given angle
hypotenuse
the side of a right triangle opposite the correct angle
unit circle
a circumvolve with a center at\,\left(0,0\right)\,and radius ane

Source: https://opentextbc.ca/algebratrigonometryopenstax/chapter/right-triangle-trigonometry/

Posted by: beckempting2000.blogspot.com

0 Response to "How To Find Acute Angle Of A Right Triangle"

Post a Comment

Subscribe to: Post Comments (Atom)

Iklan Atas Artikel

Iklan Tengah Artikel 1

Iklan Tengah Artikel 2

Iklan Bawah Artikel